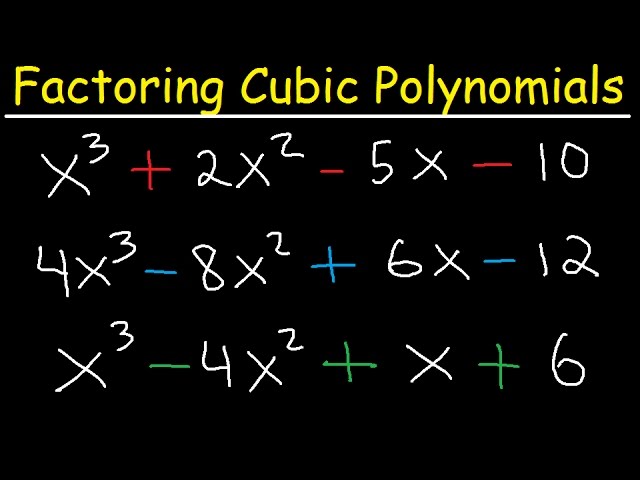
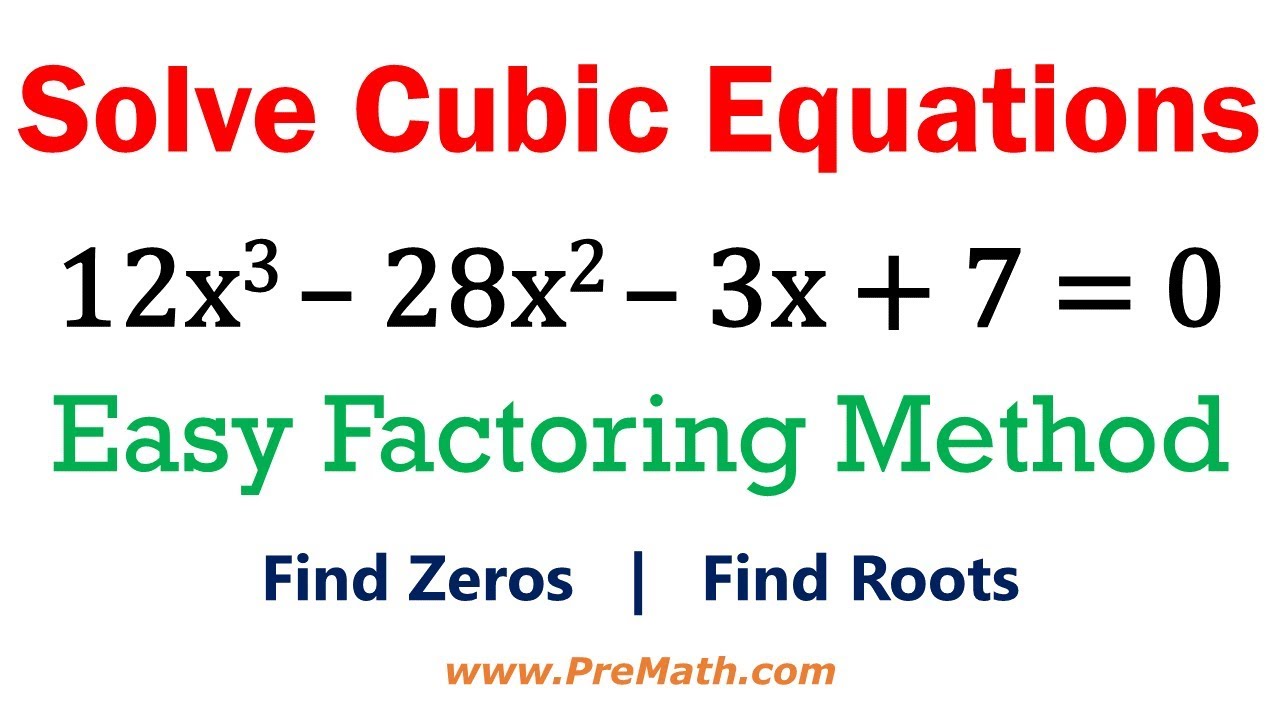
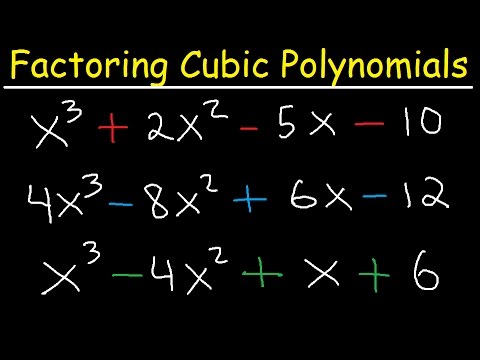
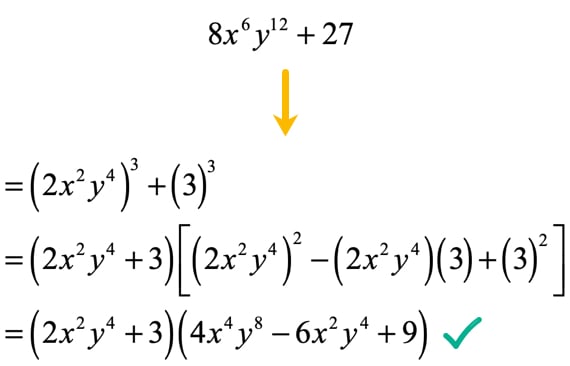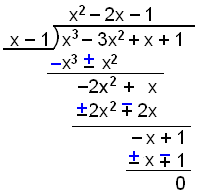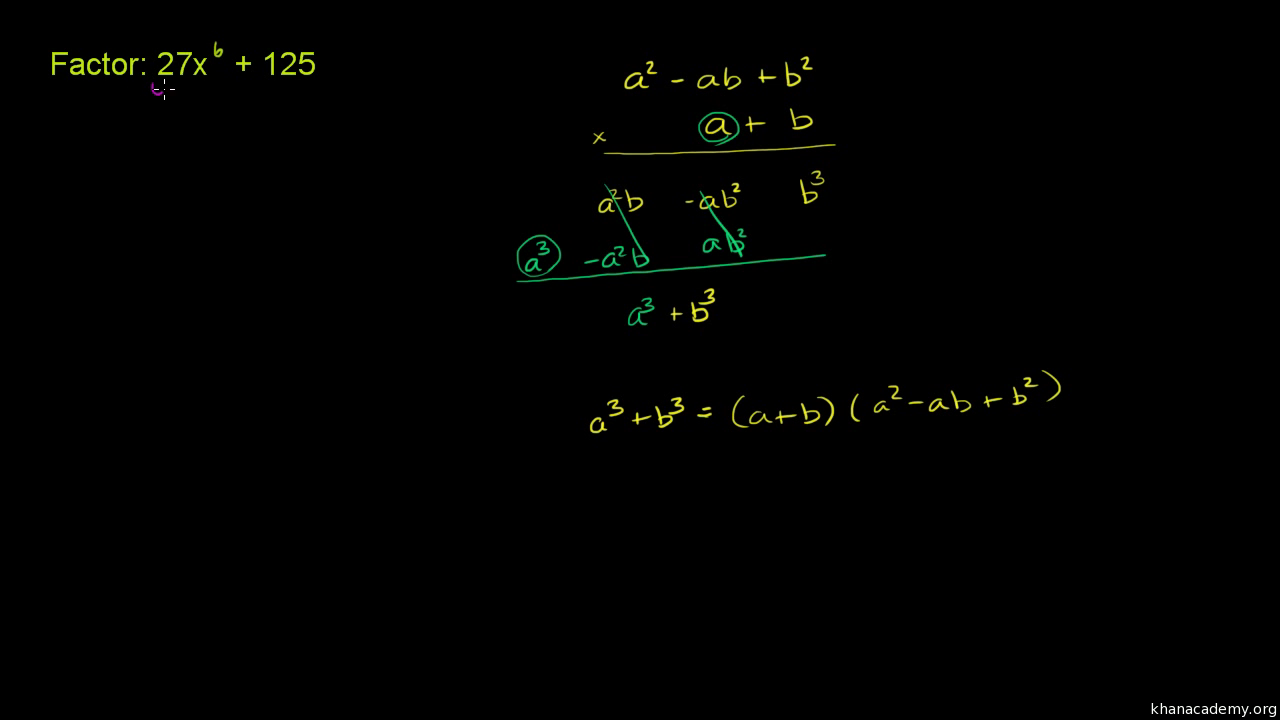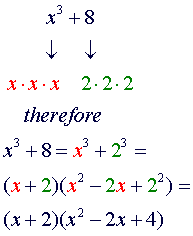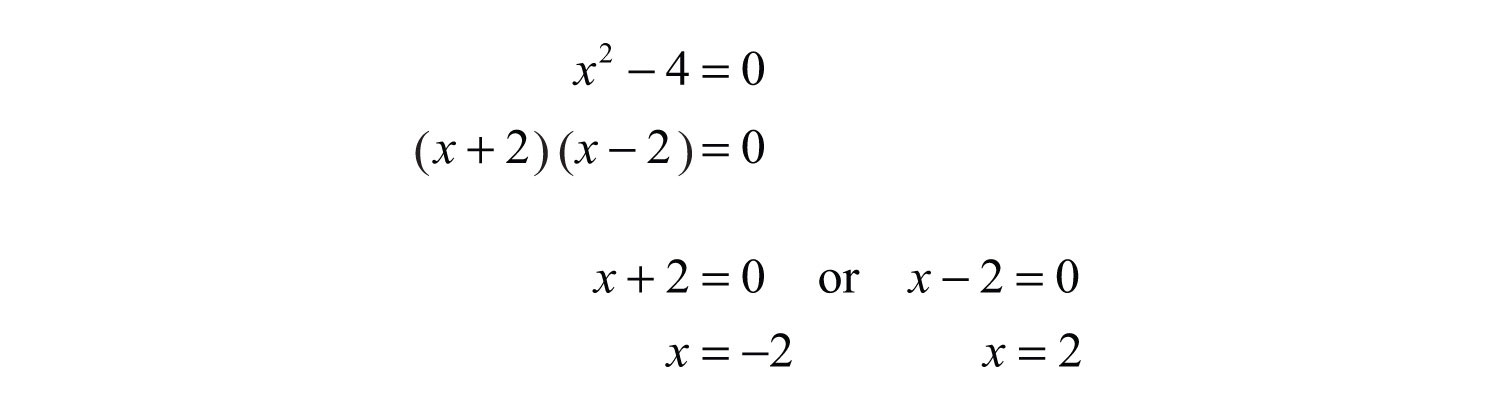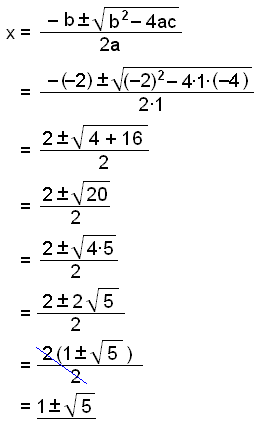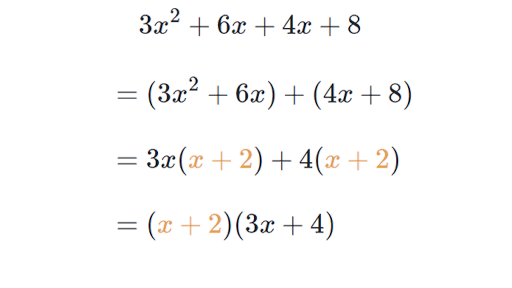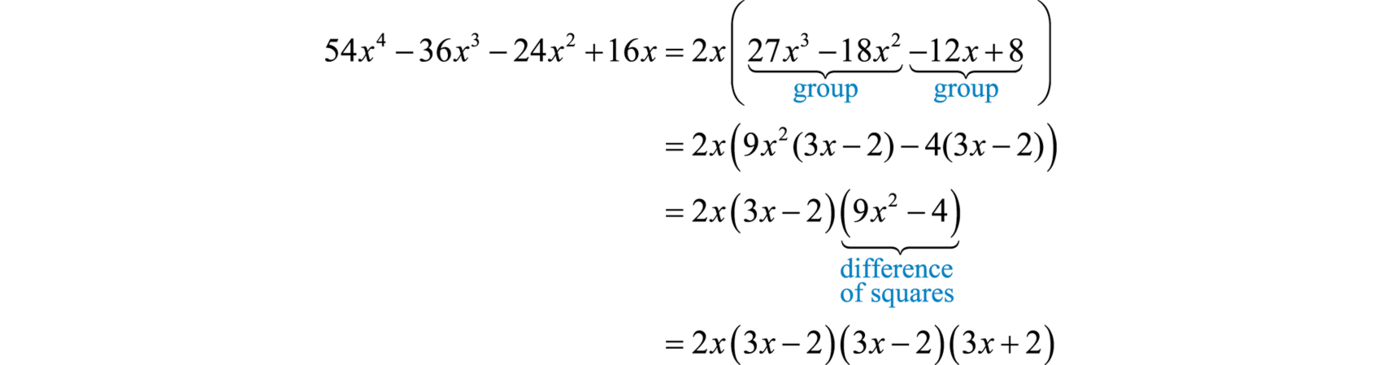How To Factor Cubic Polynomials With 4 Terms

A polynomial refers to anything with a degree or highest exponent above 2 but usually means at least 4. After having gone through the stuff given above we hope that the students would have understood how to factor polynomials with 4 terms. Provide examples if necessary.
how to factor cubic polynomials with 4 terms

Let us look into some example problems to understand the above concept.

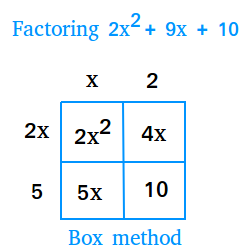
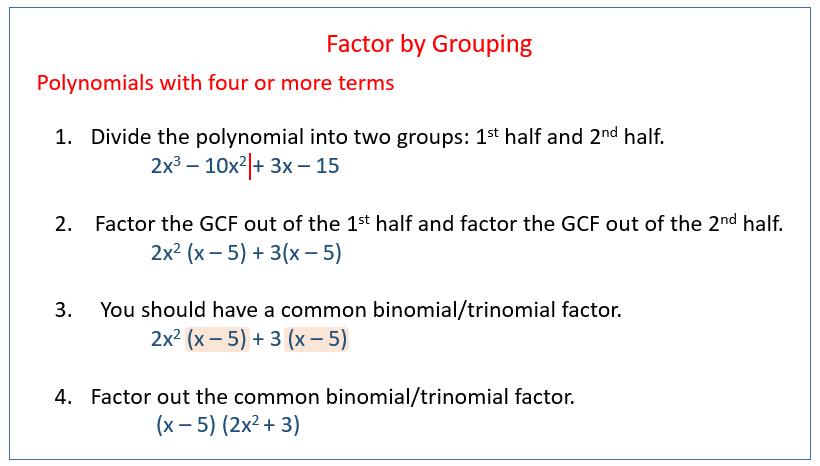
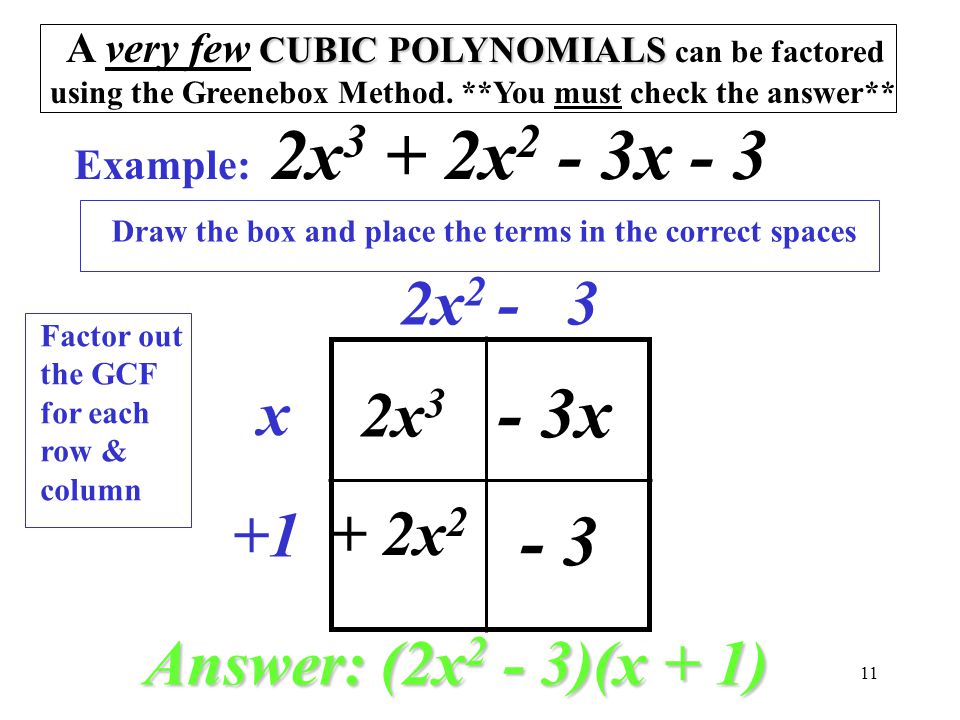
How to factor cubic polynomials with 4 terms. A polynomial of four terms known as a quadrinomial can be factored by grouping it into two binomials which are polynomials of two terms. To factor polynomials with 4 terms without grouping we use trial and error. So x 3 is a factor. Finally solve for the variable in the roots to get your solutions.

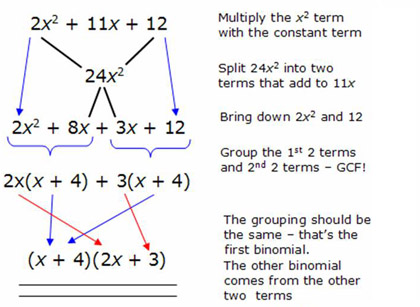
Identify and remove the greatest common factor which is common to each term in the polynomial. Trial and error means we should apply the values like 1 1 2 2 3 3etc. How to factor polynomials with 4 terms. You can go with x 3 x 2 x 1.

27 27 3 3. To factor a cubic polynomial start by grouping it into 2 sections. Some of the polynomials youll see most often are cubic polynomials or expressions with a cube as their highest variable. For example the greatest common factor for the polynomial 5x2 10x is 5x.

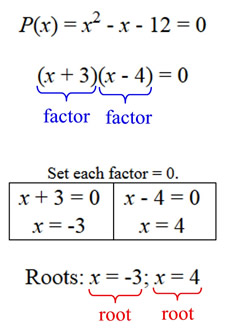
If each of the 2 terms contains the same factor combine them. P 3 33 3 32 3 3. Just follow these steps. For example if we get 0 as remainder by applying the value x 1 we may decide that x 1 is a factor.

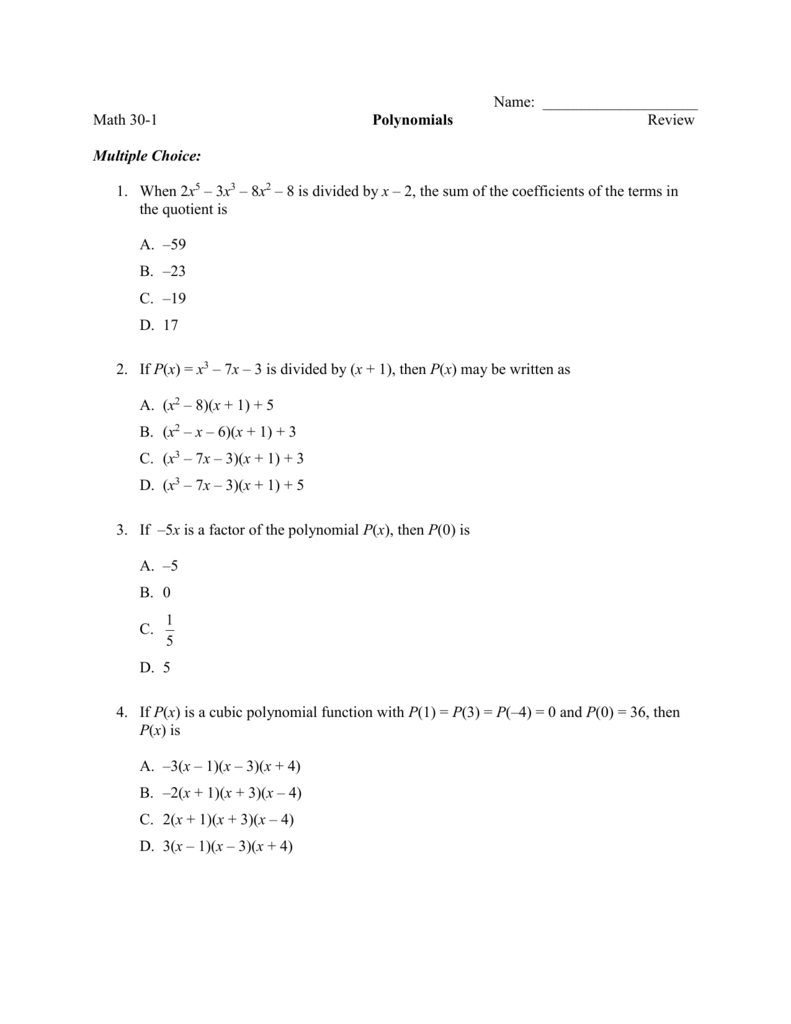
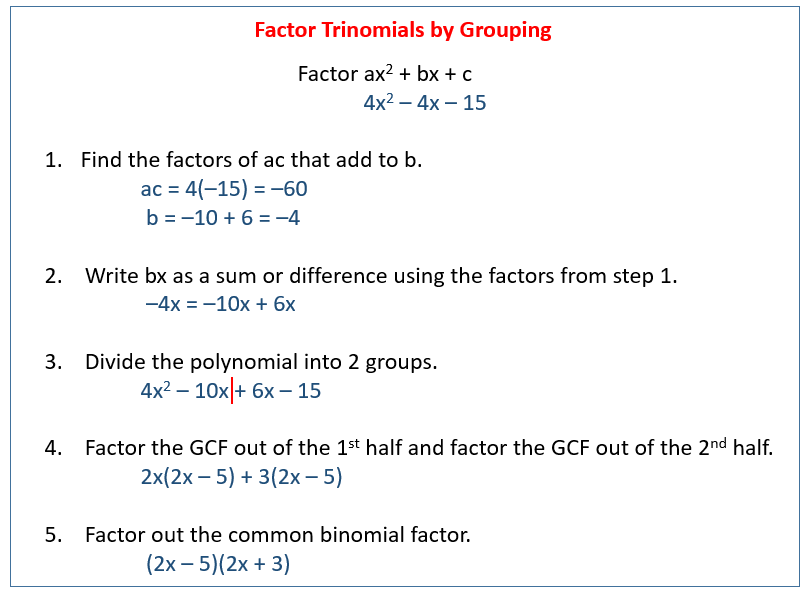
A cubic polynomial is the one which has highest degree of the variable 3. The square x 2 is the gcf of the first set and 1 is the gcf of the second set. Hence the factors are x 1 x 1 x 3. Put the plus sign between the sets just like when you.

Break up the polynomial into sets of two. Find the gcf of each set and factor it out. Polynomials are algebraic expressions that have a higher degree than the standard x 3 or y 2. Then find whats common between the terms in each group and factor the commonalities out of the terms.